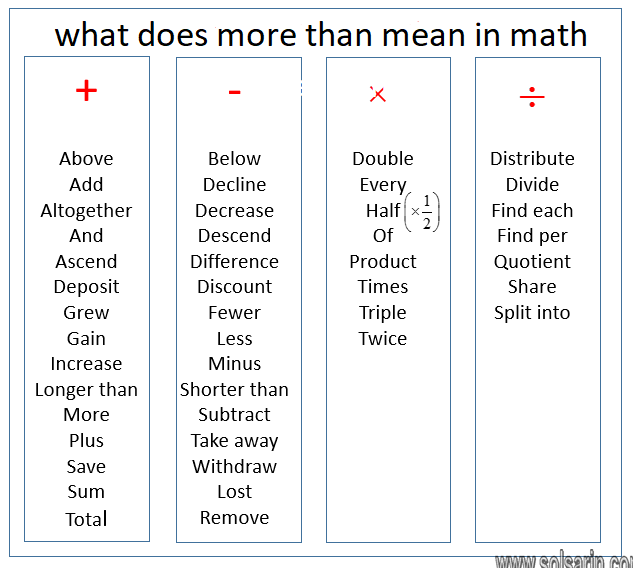
What Does More Than Mean In Math

What Does More Than Mean In Math

In this post on Solsarin we’re mentioning “what does more than mean in math”

+ Addition, Plus, Positive

The addition symbol + is usually used to indicate that two or more numbers should be added together, for example, 2 + 2.

The + symbol can also be used to indicate a positive number although this is less common

___________________________________________________________________________________

− Subtraction, Minus, Negative

This symbol has two main uses in mathematics:

1. − is used when one or more numbers are to be subtracted, for example, 2 − 2.
2. The − symbol is also commonly used to show a minus or negative number, such as −2.

_________________________________________________________________

× or * or . Multiplication

These symbols have the same meaning; commonly × is used to mean multiplication when handwritten or used on a calculator 2 × 2, for example.

The symbol * is used in spreadsheets and other computer applications to indicate a multiplication, although * does have other more complex meanings in mathematics.

Less commonly, multiplication may also be symbolised by a dot . or indeed by no symbol at all. For example, if you see a number written outside brackets with no operator (symbol or sign), then it should be multiplied by the contents of the brackets: 2(3+2) is the same as 2×(3+2).

___________________________________________________________________________________

÷ or / Division

These symbols are both used to mean division in mathematics. ÷ is used commonly in handwritten calculations and on calculators, for example, 2 ÷ 2.

/ is used in spreadsheets and other computer applications.

___________________________________________________________________________________

= Equals

The = equals symbol is used to show that the values on either side of it are the same. It is most commonly used to show the result of a calculation, for example 2 + 2 = 4, or in equations, such as 2 + 3 = 10 − 5.

You may also come across other related symbols, although these are less common:

• ≠ means not equal. For example, 2 + 2 ≠ 5 – 2. In computer applications (like Excel) the symbols <> mean not equal.
• ≡ means identical to. This is similar to, but not exactly the same as, equals. Therefore, if in doubt, stick to =.
• ≈ means approximately equal to, or almost equal to. The two sides of a relationship indicated by this symbol will not be accurate enough to manipulate mathematically.

___________________________________________________________________________________

< Less Than and > Greater Than

This symbol < means less than, for example 2 < 4 means that 2 is less than 4.

This symbol > means greater than, for example 4 > 2.

≤ ≥ These symbols mean ‘less than or equal to’ and ‘greater than or equal to’ and are commonly used in algebra. In computer applications <= and >= are used.

≪ ≫ These symbols are less common and mean much less than, or much greater than.

___________________________________________________________________________________

± Plus or Minus

This symbol ± means ‘plus or minus’. It is used to indicate, for example, confidence intervals around a number.

The answer is said to be ‘plus or minus’ another number, or in other words, within a range around the given answer.

For example, 5 ± 2 could in practice be any number from 3 to 7.

___________________________________________________________________________________

∑ Sum

The ∑ symbol means sum.

∑ is the Greek capital sigma character. It is used commonly in algebraic functions, and you may also notice it in Excel – the AutoSum button has a sigma as its icon.

___________________________________________________________________________________

° Degree

Degrees ° are used in several different ways.

• As a measure of rotation – the angle between the sides of a shape or the rotation of a circle. A circle is 360° and a right angle is 90°.
• A measure of temperature. Degrees Celsius or Centigrade are used in most of the world (with the exception of the USA). Water freezes at 0°C and boils at 100°C. In the USA Fahrenheit is used. On the Fahrenheit scale water freezes at 32°F and boils at 212°F.

___________________________________________________________________________________

∠ Angle

The angle symbol ∠ is used as shorthand in geometry (the study of shapes) for describing an angle.

The expression ∠ABC is used to describe the angle at point B (between points A and C). Similarly, ∠BAC would be used to describe the angle of point A (between points B and C).

___________________________________________________________________________________

√ Square Root

√ is the symbol for square root. A square root is the number that, when multiplied by itself, gives the original number.

For example, the square root of 4 is 2, because 2 x 2 = 4. The square root of 9 is 3, because 3 x 3 = 9.

___________________________________________________________________________________

ⁿ Power

A superscripted integer (any whole number n) is the symbol used for the power of a number.

For example,3², means 3 to the power of 2, which is the same as 3 squared (3 x 3).

4³ means 4 to the power of 3 or 4 cubed, that is 4 × 4 × 4.

Powers are also used as a shorthand way to write large and small numbers.

Large numbers

10⁶ is 1,000,000 (one million).
10¹⁰⁰ written long-hand would be 1 with 100 0’s (one Googol).

Small numbers

10^-3 is 0.001 (one thousandth)

10^-6 is 0.000001 (one millionth)

Powers can also be written using the ^ symbol.

10^6 = 10⁶ = 1,000,000 (one million)

___________________________________________________________________________________

More Posts:

What is Greater Than Sign?

The greater than sign is a mathematical symbol used to denote an inequality between two variables or quantities. This sign has been in use since the 1560s. The sign normally resembles equal – length strokes connecting in acute angle (>).

The symbol is usually placed between two quantities being compared, and it normally shows that the first variable is bigger than the second variable.
The greater than sign has been used in computer programming languages to perform other operations

For example, 2 > 1 and 1 > −2. This indicates that 2 is greater than 1 and 1 is greater than negative two.

Some of the examples greater than sign are:

5 > 2: This inequality shows that 5 is greater than 2

45 > 30: 45 is greater than 30

10/2 > 6/3: We can simplify this inequality as 5 > 2: which implies that 5 is greater than 2

0.01 > 0.001 implies that 0.01 is greater than 0.001

2 > -2: In this case, it obvious that positive numbers are greater than negative numbers. Therefore 2 is greater than – 2.

How to Remember Greater than Sign?

There are 3 methods to remember the Greater than sign.

The alligator method of remembering greater than symbol

The alligator method is the simplest technique for remembering the greater than symbol. Always remind yourself of the alligator when comparing variables using the greater than symbol.
The alligator’s mouth is always wide open to swallow or gulp as much food as possible. The mouth of the alligator usually opens to the left.

The open ends method of remembering greater than symbol

Another easy way to recall the greater than is to remember that open ends of the sign normally face the bigger number, and the arrow points to the smaller number.

L Method

In this method, recall that the less than starts with the letter L resembles the less than symbol, whereas the greater than symbol does not resemble and sign, therefore because the greater than sign does not look like an L, it cannot there be “less than.”

Solving Greater than Problems

Before attempting to solve any problem pertaining greater than symbol, the following considerations are made:

• Go through the entire question to understand it.
• Highlight the keywords to help in solving the problem
• Identify the variables
• Write the mathematical expression of the problem using the inequality symbol.
• Justify the expression

How To Write Algebraic Expressions For Word Phrases, By Analyzing The Language Used?

An algebraic expression is a mathematical phrase that contains a combination of numbers, variables and operational symbols.
A variable is a letter that can represent one or more numbers.

How to write expressions with variables?

Examples:
Write the algebraic expressions to represent the statements.
a) The sum of -7 and the quantity 8 times x
b) Take the quantity -3 times x and then add 1.
c) -6 plus the product of -1 and x.

How to Write Equations from Word Problems?

This video teaches how to dissect a word problem in order to define a variable and write an equation.

Examples:

1. Half of a number is 16. Write an equation to represent the situation. Define your variable and solve.
2. Mrs. Gaddie has two dogs. Her friend Anna-Marie has three less than twice as many as Mtr. Gaddie. How many dogs does Anna-Marie have?
3. A recycling plant recycles 2 tons of cans yesterday. This is a third of their usual amount. How much does the plant usually recycle?

How to Write Algebraic Expressions for Situations?Example:
Translate the following phrases into algebraic or numeric expressions.
a) 173 less than a number ‘b’
b) Quotient of 173 and ‘b’
c) ‘b’ times 173
d) 173 more than ‘b’

The “Greater than or equal to” symbol is used in linear inequalities when we don’t know whether the value of a variable is greater than or equal to a particular value. It is expressed by the symbol ” ≥ “.
This symbol is nothing but the “greater than” symbol ( >) with a sleeping line under it. The sleeping line below the greater sign means “equal to”.
Here is an example for you to understand this concept better. For a school to participate in an olympiad exam, the number of students from each class should be a minimum of 10.

This means that if there are less than 10 students participating from any one of the classes, none of the students of that class can take the olympiad exam.
If x represents the number of students participating in a class, then x should be greater than or equal to 10. This is represented by: x ≥ 10

Here are some other examples for “Greater than or equal to”

• x ≥ 100 means the value of x should be greater than or equal to 100.
• a ≥ – 2 means the value of a should be greater than or equal to -2.